クラスカルウォリス検定は、ノンパラメトリック版の一元配置分散分析
平均値というパラメータを使わないが、その結果はどのように書いたらよいか
クラスカルウォリス検定はどんな計算をしているのか
クラスカルウォリス検定は、連続データを順位に変換して、平均順位を比較するという方法で計算されている
詳しくはこちらを参照


クラスカルウォリス検定の結果の書き方
では、論文では、クラスカルウォリス検定の結果はどのように書かれているだろうか
p 値以外に、何を書いたらよいか悩むという意味である
クラスカルウォリス検定の検定統計量の計算式から考えれば、平均順位が適切とは思うが、実際はどうだろうか
平均値 SD 中央値
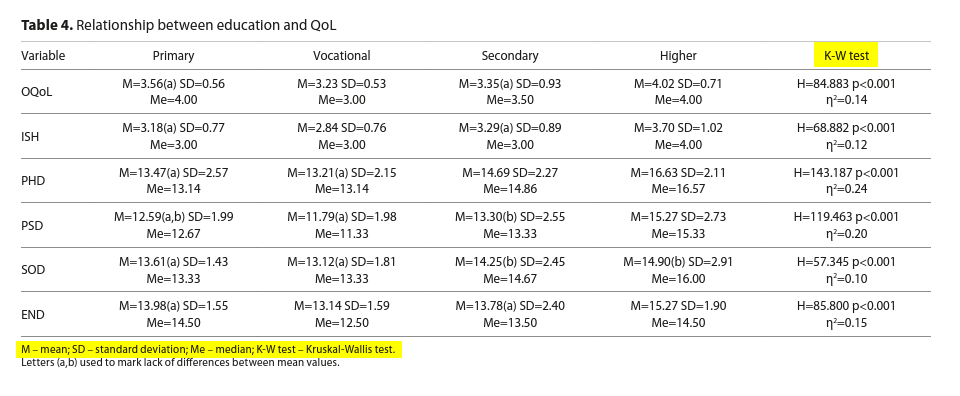
平均値±SD
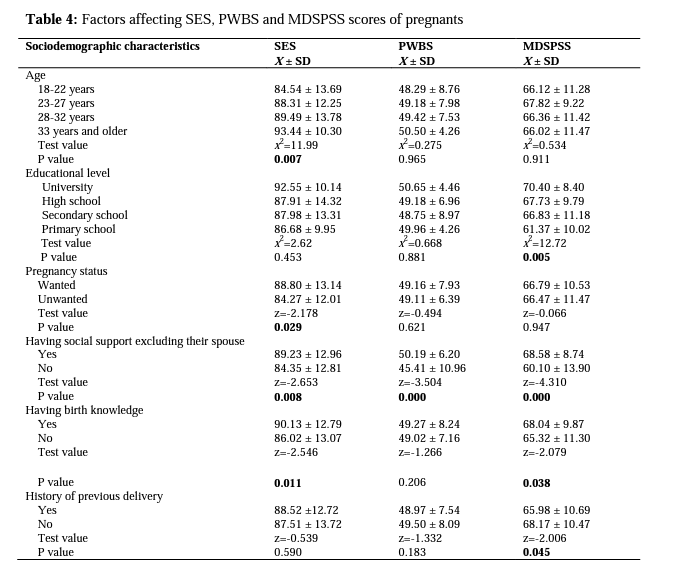
Self-efficacy, Psychological Well-Being and Perceived Social Support Levels in Pregnant Women
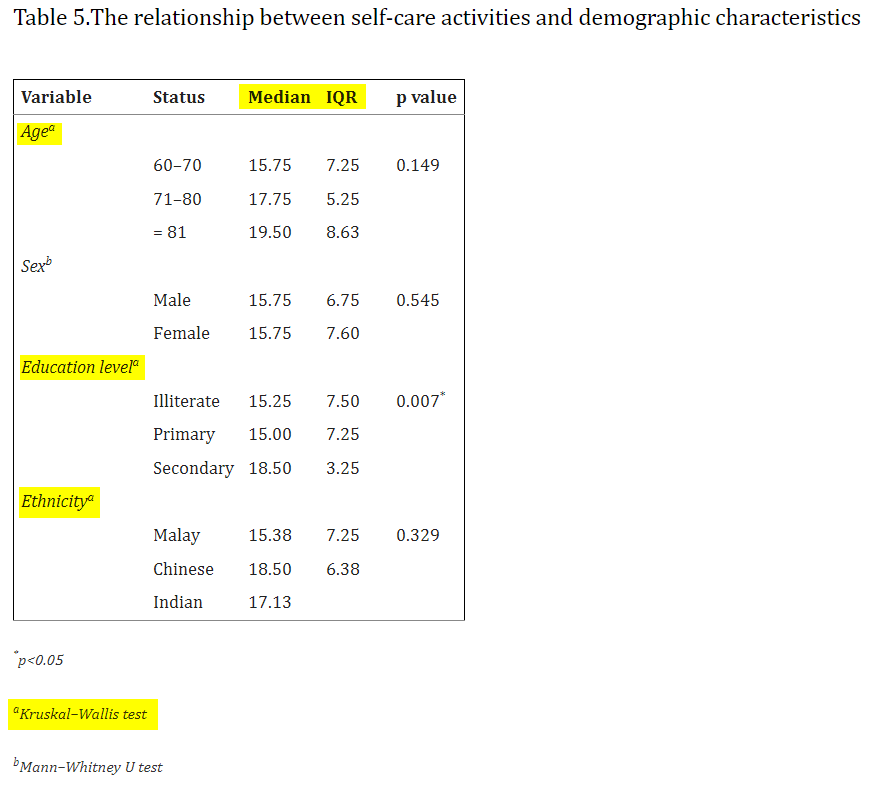
中央値及び四分位範囲
Social support and self-care activities among the elderly patients with diabetes in Kelantan – PMC
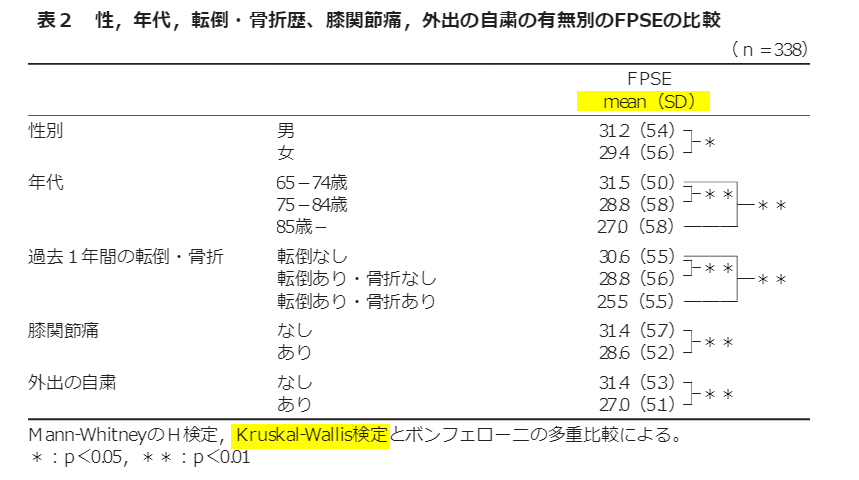
平均順位
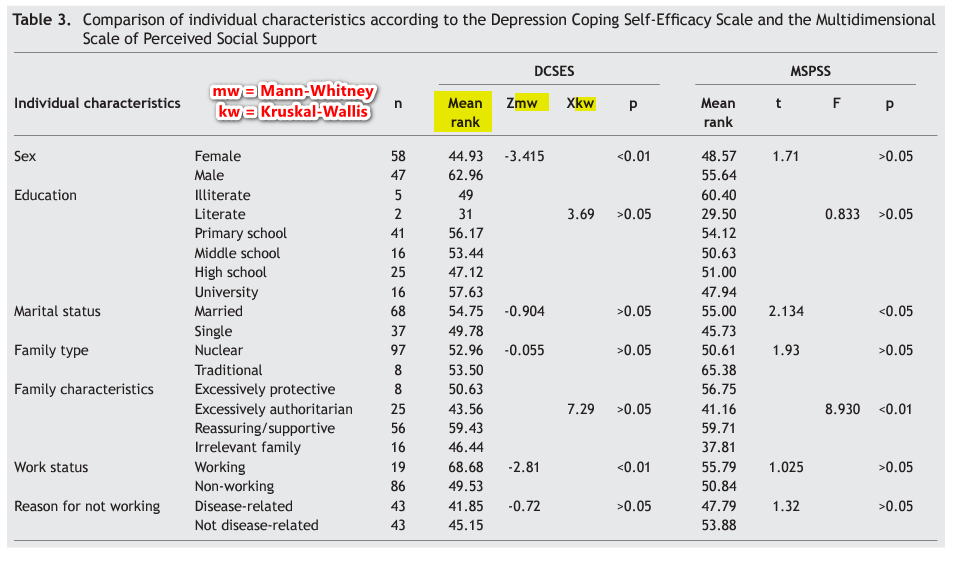
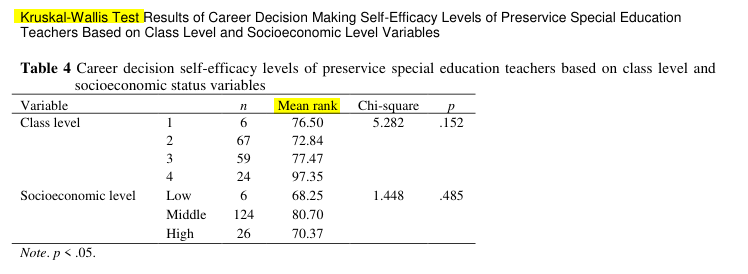
ということで、なぜか使わないはずの平均値が示されていたり、中央値が示されていたり、計算とは全く関係ない代表値が書かれていることがわかる
平均順位で示されている論文も散見されたが、少数派の印象がある
理屈で考えると変かもしれないが、現実に公表されている数値を真似するのが得策である
まとめ
クラスカルウォリス検定の結果の書き方を、公表されている論文で確認してみた
p 値以外は、平均値、標準偏差、中央値、四分位範囲などよく知られている代表値、ばらつきの指標が示されていることがわかる
これらを真似するのが良いと思う
参考になれば
参考論文
Self-efficacy, Psychological Well-Being and Perceived Social Support Levels in Pregnant Women




コメント